本文共 2844 字,大约阅读时间需要 9 分钟。
前面的章节已经提到过,Storm集群中的任务称之为Topology。
对比Hadoop中的MapReduce job,mapper用于从数据源中获取数据,经过简单处理,以一定的格式传递给reducer,reducer负责后续处理。
类似的,Topology任务也要从数据源中获取数据,然后进行后续处理。在Topology中从外部数据源获取数据的组件,称之为Spout,处理数据的组件,称之为bolt。一个Topology就是由一个或者多个的Spout和Bolt组成。
对于如下:
数据获取 数据处理
Hadoop Mapper Reducer Storm Spout Bolt 小贴士:Storm中有一些术语(不是全部),是按照气象术语来命名的。例如Storm是暴风雨,spout是龙卷风的意思,bolt是雷电的意思,nimbus是雨云的意思。接下来,我们从简单到复杂逐步分析一个Topology的组成。
1、最简单的Topology:1个Spout,1个Bolt

Topology:在这个Topology中,我们看到一个Spout和一个Bolt。在Topology中,我们将Spout和Bolt称之为组件(Components)。一个Topology中,必须同时存在Spout和Bolt,Spout和Bolt数量可以随意。最简单的就是如上图,一个Topology中只有一个Spout和一个Bolt。要提醒的是:Topology的组件目前只有Spout和Bolt,没有其他组件。所以以后提到一个Topology的组件的时候,其实也就是指的是Spout或者Bolt。
Stream:我们已经知道Spout是从外部数据源中获取数据,以一定的格式将数据传递给Bolt处理。从Spout中源源不断的给Bolt传递数据,形成的这个数据通道我们称之为Stream(流)。因为Strom是一个实时计算的流式处理框架,其不是像hadoop那样,一次性处理一大批的数据(批处理),Storm是不断从外部数据源中获取最新的数据,然后将新的数据传递给Bolt处理(增量处理)。这样不断的获取与传输就形成了这个数据流通道就称之为Stream,如上图的虚线所示。
Tuple:Tuple这个概念在上面没有明显的标记出来,其实Stream的最小组成单元。上图中的Steam是一条虚线,我们可以将虚线中的每一个小线段"-“看成一个Tuple。因为Storm的Spout需要源源不断的向Bolt发送数据,每一次发送的数据我们称之为一个Tuple,也就是说,Stream流实际上就是通过这些源源不断的Tuple组成。在Storm官网上有这样一句话” A stream is an unbounded sequence of tuples …",unbounded的意思其实就是说,Spout是源源不断的发送Tuple给Bolt的,我们不知道什么时候会停止发送Tuple,因此当然是无边界的。
2、稍微复杂的Topology:1个Spout、多个Bolt
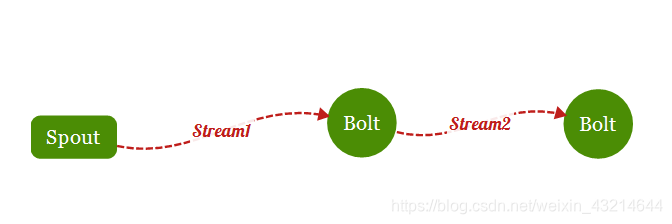
Topology:在这个Topology中,我们有一个spout和3个bolt。从数据流向中,我们可以看出,spout可以将数据传递给多个bolt,bolt还能将数据传递给下一级bolt。我们知道Bolt在Topology中的作用是处理器,也就是说Bolt是负责处理数据的。对于同一条数据,我们可能会挖掘出不同方面的信息。以中间的并列的2个Bolt为例,Spout可以将数据同时发送给2个Bolt,这两个Bolt分别从不同的方便来分析这条数据。我们还可以看到,位于中上方的Bolt还将数据传递给了下一级Bolt,这样的原因是,有的时候,我们对于数据的分析必须是一步一步的,后一步的分析必须建立在之前分析的基础上。
Stream:在上图中,我们标记出了3个Stream。在Topology中,Spout与Bolt,Bolt与Bolt之间的数据通道都是一个流。图上分别用Stream1,Stream2、Stream3标记出。这三个流有什么区别和联系吗?必须的。我们已经知道,Stream都是由Tuple组成的,而Tuple是有数据格式的,在同一个流中,Tuple的数据格式应该都是一样的;不同流中的数据格式可能相同,也可能不同。举例来说,假设我们这里的Spout每次都是将相同的数据发送给中间的2个Bolt进行处理,那么Stream1和Stream2中Tuple的数据格式都是一样的。前一个Bolt处理完还要将数据传递给下一级Bolt,处理完后的数据可能是另外一种格式。这时Stream3数据流中的数据格式可能与Stream1和Stream2就是不同的了。
3、复杂的Topology:多个Spout与多个Bolt
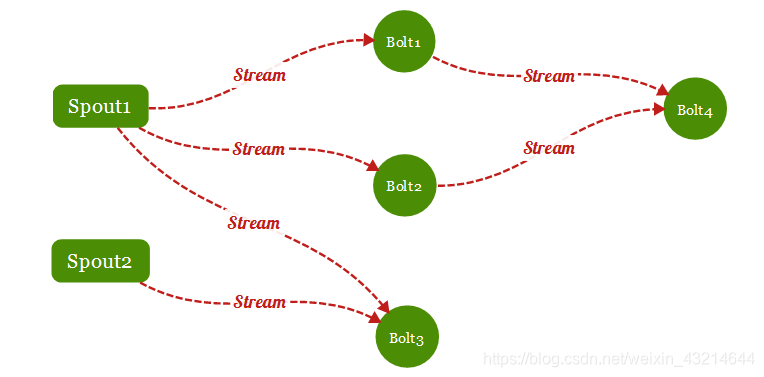
Topology:这可以算得上一个比较复杂的Topology了,在图中有2个Spout、4个Bolt。再次深入,我们发现一个Spout可以同时发送数据到多个Bolt,形成多个数据流,例如Spout1。一个Bolt可以接受多个输入,如bolt3、bolt4。事实上,一个Bolt也可以产生多个到下一级Bolt的数据流,在图中并没有体现出来。
我们在这里主要介绍一下,什么情况下一个Topology中会需要多个Spout。以一个实际案例,假设有一个移动医疗app。用户可以在APP上进行问诊,会有医生来进行回答。这种情况下,医生头像在APP上显示的位置必须是实时变化的,因为如果咨询同一个医生的用户太多,后面的用户就会排队很长时间。因此,我们会使用Storm来实时计算医生的权重,权重越高,说明这个医生当前比较空闲,而权重越低,说明医生越忙。APP会实时获取医生权重,将权重高的医生,排在越前面。现在的问题就是如何计算医生的权重,简单说2个维度:问诊量、排队数。问诊量表示的是当前医生同时回答的病人的数量,排队数是有几个人在排队。基本上问诊量越低,排队数越少,说明这个医生越闲,权重应该就越高。在我们的Topology中,就可以用两个Spout,一个用于从外部数据源中获取医生的问诊量,一个用于获取排队数。当然实际的情况比这个肯定复杂,这里只是说明多个Spout在什么情况下会使用到。
DAG(有向无环图)说明:
在storm中,spout和bolt、bolt与bolt之间的数据流向,将整合topology串起来了。在网上千篇一律的翻译或者转载的文章中,我们可能也看到了很多次DAG这个概念。其实DAG很简单,有向无环图,意思就是数据流是有方向的,但是不能形成一个环状。如果形成了一个环状,那么意味着Bolt中的数据还可能传给Spout,spout又要传递给Bolt。这就形成了一个死循环,Stream中的一个数据(Tuple)永远也没办法处理完。
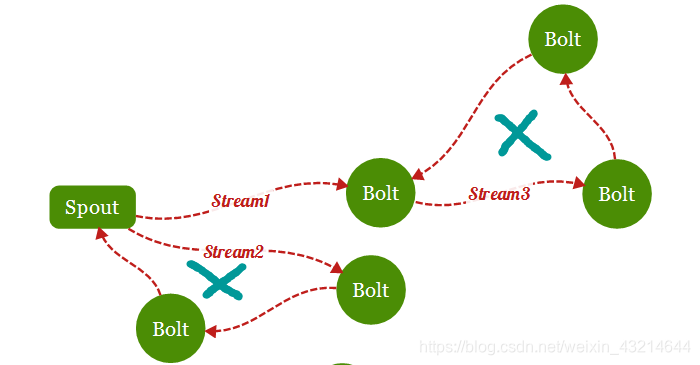
以下这个图有2个环,不论是右上Bolt之间形成的环,还是右下Spout与Bout之间形成的环,都是不允许。